Then we need to see whether the problem wants us to use the n th term formula or the sum of n terms formula. To use the sequence formulas, first, we need to identify whether it is arithmetic or a geometric sequence. The geometric sequence formulas are used further to deduce compound interest formulas. The sequence formulas are used to find the n th term (or) sum of the first n terms of an arithmetic or geometric sequence easily without the need to calculate all the terms till the n th term. What Are the Applications of Sequence Formulas? In the same way, n th term = a + (n - 1) d. If we observe the pattern here, the first term is a = a + (1 - 1) d, the second term is a + d = a + (2 - 1) d, third term is a + 2d = a + (3 - 1) d. i.e., it is of the form a, a + d, a + 2d. In an arithmetric sequence, the difference between every two consecutive terms is constant.
How To Derive n th Term of an Arithmetic Sequence Formula? The sequence formulas related to the geometric sequence a, ar, ar 2. The sequence formulas related to the arithmetic sequence a, a + d, a + 2d. They mainly talk about arithmetic and geometric sequences. The sequence formulas are about finding the n th term and the sum of 'n' terms of a sequence.
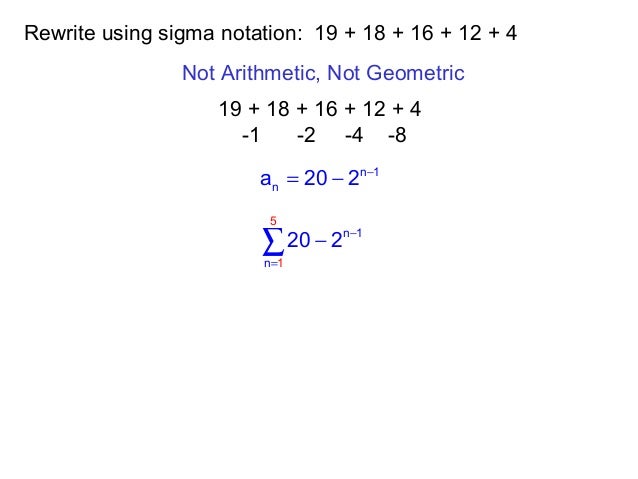n th term of arithmetic sequence (implicit formula) is, \(a_n\) = \(a_\) = 1 (-3) 15 - 1 = (-3) 14 = 4,782,969Īnswer: The 15 th term of the given geometric sequence = 4,782,969.įAQs on Sequence Formula What Are Sequence Formulas?. Note how only whole numbers are used, because it doesn't. If a formula is provided, terms of the sequence are calculated by substituting n0,1,2,3. n th term of arithmetic sequence (explicit formula) is, \(a_n\) = a + (n - 1) d. Geometric sequences are formed by choosing a starting value and generating each subsequent value by multiplying the previous value by some constant called the geometric ratio. Here are the formulas related to the arithmetic sequence. where the first term is 'a' and the common difference is 'd'. Let us consider the arithmetic sequence a, a + d, a + 2d. Example 2.2.3 Find the recursive and closed formula for the sequences below. Then we have, Recursive definition: an ran 1 with a0 a. Suppose the initial term a0 is a and the common ratio is r. Here are the sequence formulas which will in detail be explained below the list of formulas. Geometric Sequences A sequence is called geometric if the ratio between successive terms is constant. The sequence formulas include the formulas of finding the n th term and the sum of the first n terms of each of the arithmetic sequence and geometric sequence. Let us learn the sequence formulas in detail along with a few solved examples here. A geometric sequence is a sequence in which the ratio of every two consecutive terms is constant. An arithmetic sequence is a sequence in which the difference between every two consecutive terms is constant. We have two types of sequence formulas, arithmetic sequence formulas, and geometric sequence formulas.
0 kommentar(er)
